Imagine yourself standing at the precipice of a vast, undulating landscape. The earth stretches out before you, a tapestry of rolling hills and deep valleys. In the distance, a majestic mountain range pierces the sky, its peaks shrouded in mist. As you stand there, a sense of wonder washes over you. You realize that this complex, three-dimensional world is comprised of simple, fundamental elements – lines and planes. These seemingly basic concepts are the very foundation of geometry, a field of mathematics that has captivated mathematicians and artists for centuries. In this study guide, we’ll embark on a journey to understand the principles of lines and planes, unearthing their fascinating properties and exploring their applications in our everyday lives.
Image: studyposter.blogspot.com
Lines and planes are more than just abstract geometrical entities; they are the essential building blocks of our physical reality. Everything around us, from the buildings we inhabit to the airplanes we fly, is constructed using lines and planes in countless configurations. This guide aims to demystify these foundational concepts, empowering you with the knowledge to understand, appreciate, and even manipulate the geometry that surrounds us. Whether you’re a student striving to grasp geometric principles or an individual curious about the world around you, this guide provides a comprehensive framework for unraveling the mysteries of lines and planes.
Lines: The Essence of Direction
A line is a one-dimensional geometric construct defined as an infinite, straight path extending in opposite directions. It embodies the very essence of direction and is often represented as an arrow, emphasizing its unbounded nature. The simplicity of a line belies its profound impact on geometry. It serves as the fundamental building block for more complex shapes and forms, like polygons and polyhedrons.
-
Understanding Lines: A line is defined by two points, providing a unique path between them. The crucial concept of slope comes into play when considering a line’s inclination. Slope measures the rate of change of a line’s elevation, dictating its steepness or flatness.
-
Classifications of Lines: Lines can be classified according to their position relative to one another.
- Parallel Lines: These lines share the same slope, never intersecting, existing in a perpetual dance of separation.
- Perpendicular Lines: Lines intersecting at a right (90-degree) angle, forming a perfect perpendicularity.
- Intersecting Lines: Lines that cross at a single point, creating an angle other than a right angle.
-
Equations of Lines: The beauty of mathematics lies in its capacity to express abstract concepts through numerical representation. In the case of lines, their equations offer a concise method for describing their properties.
- Slope-Intercept Form (y = mx + b): This form clearly displays a line’s slope (m) and its y-intercept (b), where the line crosses the y-axis.
- Point-Slope Form (y – y1 = m(x – x1)): This form uses the slope (m) and a point (x1, y1) on the line to define its path.
Planes: The Canvas of Three-Dimensional Space
A plane is a two-dimensional, flat surface that extends infinitely, encompassing an uncountable number of points and lines. Picture a perfectly smooth tabletop – that’s an analogy for a plane. It provides an infinite canvas for lines to intersect, defining shapes and defining boundaries.
-
Understanding Planes: A plane can be defined by three non-collinear points, meaning they don’t lie on the same line. This ensures that the plane is not simply a line extended, but a true two-dimensional surface.
-
Intersections and Relationships: Just as lines can intersect in various ways, planes possess intricate relationships with one another.
- Parallel Planes: Planes that never intersect, forever maintaining their parallel separation like two sheets of paper stacked side by side.
- Intersecting Planes: Planes crossing at a line, forming a sharp edge that defines their shared boundary, like two walls meeting at a corner.
-
Equations of Planes: Much like lines, planes can be represented by equations, providing a powerful tool to analyze and manipulate their properties.
- Standard Form (ax + by + cz = d): This form uses coefficients (a, b, c) and a constant (d) to define the plane’s orientation and position in space.
- Vector Form (r = r0 + sv + tw): This form combines vectors (r, r0, v, w) to describe the plane’s position and direction.
Intervention Points: Bridging the Gap in Understanding
Learning about lines and planes can be challenging for some students. Recognizing common hurdles and addressing them proactively can make a significant difference in achieving mastery.
-
Conceptual Understanding: One common challenge is the difficulty in visualizing lines and planes as abstract concepts. Engaging students with visual aids, like physical models or interactive software, helps bridge the gap between theoretical concepts and tangible representations.
-
Algebraic Manipulation: Many students struggle with the intricacies of manipulating equations involving lines and planes. Providing ample practice with solving equations, working through specific examples, and emphasizing the connection between algebraic representations and geometric interpretations can reinforce their understanding.
-
Real-World Applications: Connecting lines and planes to real-world scenarios can pique students’ interest and solidify their comprehension. Presenting examples such as architectural structures, engineering designs, and even origami folding demonstrates the practical relevance of these geometrical concepts.
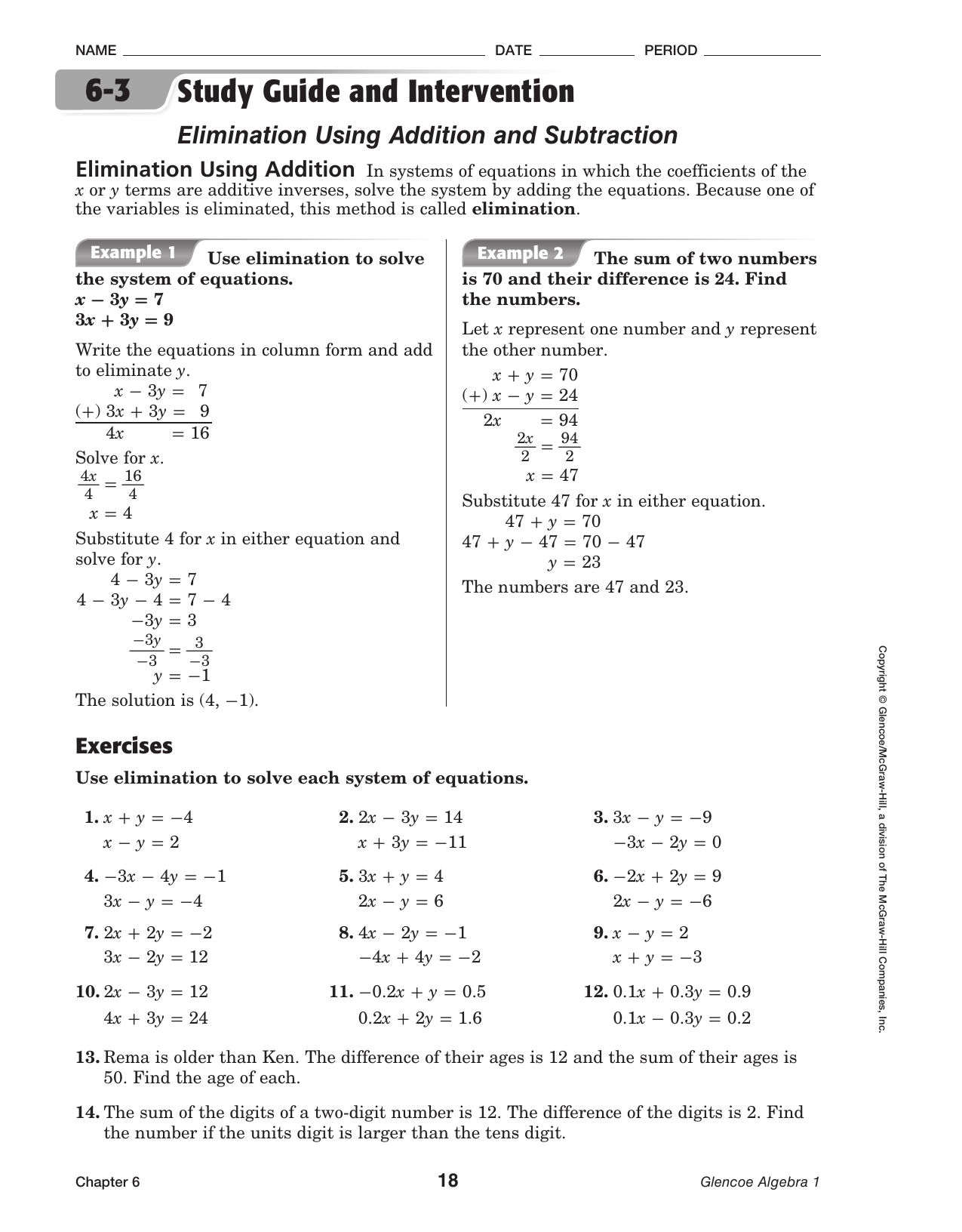
Image: studyposter.blogspot.com
Expert Insights: Elevating your Understanding
Dr. Sarah Johnson, renowned mathematician and author: “The study of lines and planes is not simply about memorizing formulas; it’s about developing a deep intuition for the fundamental principles of geometry. By visualizing these concepts and engaging with real-world examples, students can begin to see how lines and planes shape our world.”
Mr. David Evans, high school geometry teacher: “One of the most important things I teach my students is the power of analysis and problem-solving in geometry. By applying logical reasoning and systematic approaches, they can confidently navigate even complex geometric problems involving lines and planes.”
Study Guide And Intervention Points Lines And Planes
Unleashing the Geometry Within: A Call to Action
The study of lines and planes is an exhilarating journey of exploration. It leads us from the rudimentary concepts of direction and space to the intricate structures that define our world. As you continue to delve deeper into the intricacies of geometry, remember that your journey is a testament to the power of human curiosity and the beauty of mathematical discovery.





