Geometry is a fascinating subject, filled with intriguing shapes and patterns. While some shapes like circles and squares might seem relatively simple, triangles can be a bit trickier to master. Remember that fateful day in middle school when you first encountered the Pythagorean theorem? It wasn’t exactly a walk in the park! But even within the realm of triangles, there are some specific types that are easier to grasp, and these are the isosceles and equilateral triangles.
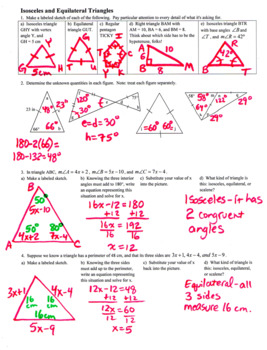
Image: www.teacherspayteachers.com
If you’re currently struggling with your geometry homework and need a little extra help with isosceles, equilateral triangles, and how to solve problems related to them, then you’ve come to the right place! This article will walk you through the principles of isosceles and equilateral triangles, provide examples to illustrate the concepts, and give you helpful tips on working through a worksheet. Plus, we’ll uncover where to find some very handy isosceles and equilateral triangles worksheet PDF answer keys!
Understanding Isosceles and Equilateral Triangles
Triangles, as you know, are closed figures with three sides and three angles. But what makes isosceles and equilateral triangles special is the relationship between their sides and angles.
Isosceles Triangles
An isosceles triangle has two sides of equal length. These equal sides are called the “legs” of the triangle, and the third side is called the “base.” Due to the symmetry inherent in this configuration, the two angles opposite the equal sides (known as the base angles) will also be equal.
Let’s break it down with a simple example. Imagine a triangle where AB = AC. This means that angle B (opposite side AC) and angle C (opposite side AB) will also be equal. Because the sum of all three angles in a triangle is always 180 degrees, we can deduce the measure of each base angle by using the formula: (180 – angle A) / 2.
Equilateral Triangles
Equilateral triangles are even more special. They have all three sides equal in length, which means all three angles are also equal! Since the sum of the angles in any triangle is 180 degrees, each angle in an equilateral triangle will always measure 60 degrees.
Understanding the properties of isosceles and equilateral triangles helps us solve numerous geometry problems. Let’s dive into some practical examples.

Image: worksheetfullkristi.z21.web.core.windows.net
Problem-Solving with Isosceles and Equilateral Triangles
There are many types of problems you might encounter when dealing with isosceles and equilateral triangles. Here are a few examples:
Example 1: Finding a Missing Angle
If you are given an isosceles triangle with one known base angle of 40 degrees, how would you find the measure of the other two angles? Remember that in an isosceles triangle, the base angles are equal. Therefore, the other base angle is also 40 degrees. The third angle in the triangle would be 180 – 40 – 40 = 100 degrees.
Example 2: Finding a Missing Side
Consider an equilateral triangle where one side is 5 cm long. Since all sides of an equilateral triangle are equal, the other two sides are also 5 cm long.
Example 3: Area and Perimeter
For isosceles and equilateral triangles, you can calculate area and perimeter using basic geometric formulas.
The perimeter of any triangle is simply the sum of the lengths of its sides. In an isosceles triangle, the perimeter would be calculated as: (2 * length of leg) + length of base. For an equilateral triangle, the perimeter would be: (3 * length of side)
The area of a triangle is calculated using the formula: (1/2) * base * height. Remember that the “height” refers to the perpendicular distance from the base to the opposite vertex, and in isosceles triangles, this height bisects the base.
Isosceles and Equilateral Triangles Worksheet PDF Answer Key: Where to Find Help
We’ve covered the basics of isosceles and equilateral triangles, but now you need to test your knowledge! Enter the indispensable isosceles and equilateral triangles worksheet PDF answer keys.
These resources are a lifeline for students wanting to check their answers and understand the solutions to problems they might be struggling with. Here are some key places to look for these answer keys:
- Your Textbook or Online Resources: Often, your geometry textbook will come with answer keys for various exercises, including those involving isosceles and equilateral triangles. Online resources such as Khan Academy, IXL, and Chegg Study can also provide valuable practice problems with answer keys.
- Tutoring Websites: There are many reputable tutoring websites like TutorMe and Skooli that offer comprehensive help with geometry, including answer keys to worksheets. These websites often have experienced tutors available to clarify any concepts you might find challenging.
- Free Online Resources: Many websites offer free downloadable worksheets for various subjects, including isosceles and equilateral triangles. Some websites also provide accompanying answer keys that you can use to check your work.
Tips for Mastering Isosceles and Equilateral Triangles
The world of geometry can be daunting, but with the right approach, you can conquer those isosceles and equilateral triangles! Here are some tips:
- Practice, Practice, Practice: The adage, “Practice makes perfect,” holds true for geometry. The more you practice working with these triangles, the more confident you’ll become.
- Visualize the Concepts: Draw diagrams to illustrate the properties of isosceles and equilateral triangles. This visualization will help solidify your understanding.
- Break Down Complex Problems: Don’t get overwhelmed by complicated problems. Break them down into smaller, more manageable steps.
- Utilize Online Tools: There are online tools that can help you visualize and solve geometry problems. Experiment with these tools to enhance your understanding.
- Ask for Help: If you struggle with a particular problem, don’t hesitate to ask your teacher, classmates, or a tutor for help. There’s no shame in seeking guidance.
Remember, geometry is not about memorizing formulas; it’s about understanding the underlying concepts and applying them to solve real-world problems. As you practice and explore, you’ll discover the beauty and logic inherent in the world of shapes and figures.
Isosceles and Equilateral Triangles: Frequently Asked Questions
You’ve learned the basics of isosceles and equilateral triangles, but you’re still curious. Here are some commonly asked questions about these shapes:
Q: Can a triangle be both isosceles and equilateral?
A: Yes! An equilateral triangle is a special case of an isosceles triangle where all three sides are equal.
Q: Can an isosceles triangle be a right triangle?
A: Yes, it’s possible. If the equal sides of an isosceles triangle are the legs of a right triangle, then it will be an isosceles right triangle.
Q: Are there any real-world examples of isosceles and equilateral triangles?
A: Absolutely! Equilateral triangles can be found in nature, such as snowflakes and the hexagonal honeycomb structure of bees. Isosceles triangles are often used in architecture and construction for their symmetrical and stable properties.
Are you starting to see the world through a geometric lens now?
Isosceles And Equilateral Triangles Worksheet Pdf Answer Key
https://youtube.com/watch?v=KiHgxHrJh3Y
Conclusion
Mastering isosceles and equilateral triangles is a crucial step in your journey through the world of geometry. From understanding basic properties to solving complex problems, we’ve covered some key points and provided helpful resources. Remember, practice, visualization, and seeking help are your best allies!
Are you ready to tackle those isosceles and equilateral triangles with newfound confidence? Let us know your thoughts, experiences, and any questions you have in the comments below!





