Remember that time in geometry class when you were introduced to the concept of surface area, and your mind was blown by the idea of measuring the outer “skin” of three-dimensional shapes? Well, let’s revisit that with a focus on spheres and hemispheres. These shapes present unique challenges, but with the right tools and understanding, calculating their surface areas becomes a breeze. So, dive in with us as we explore the captivating world of spheres, hemispheres, and the handy worksheets that help us master their surface area calculations.
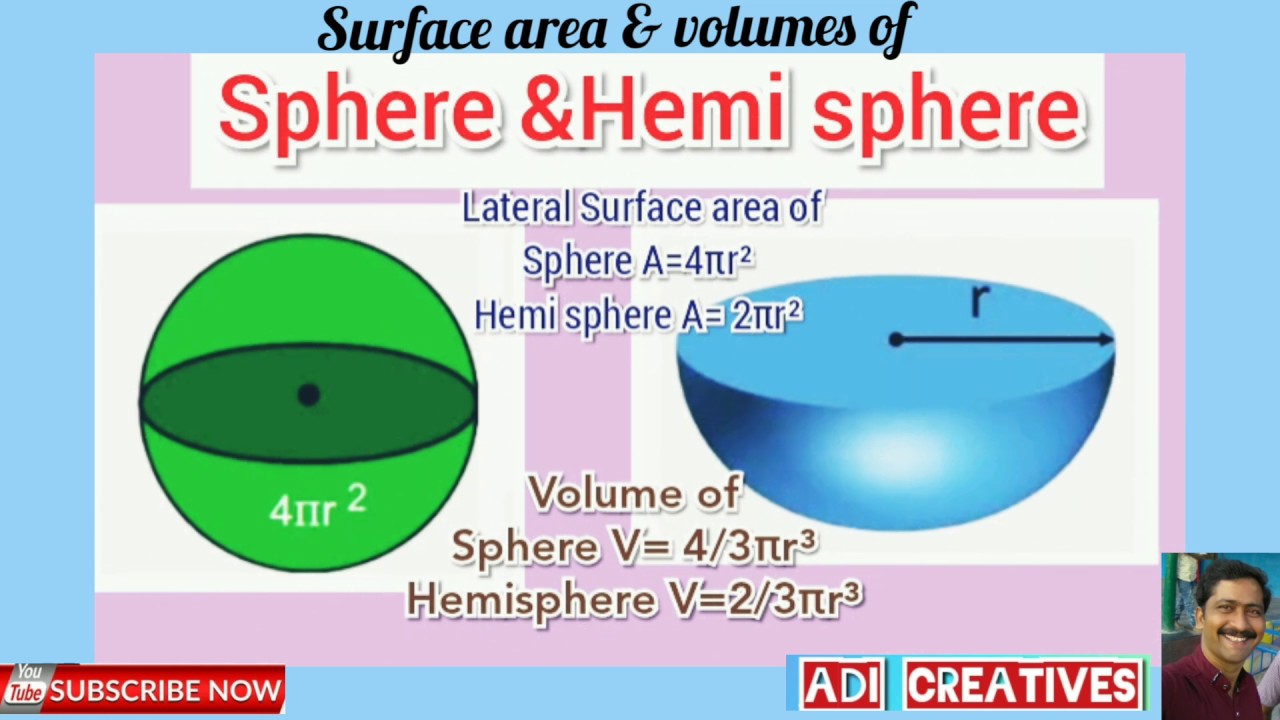
Image: lessonmagicbrandt.z4.web.core.windows.net
Navigating the complexities of sphere and hemisphere surface area calculations can seem daunting. But fret not! This article will equip you with the knowledge, formulas, and practice problems you need to conquer any worksheet, turning those seemingly complex problems into straightforward exercises. We’ll journey through the world of spherical geometry, delve into the practical applications of these concepts, and offer insights to help you tackle any challenge with confidence.
Delving into the Realm of Spheres and Hemispheres
Before we dive into the nitty-gritty of calculating surface area, let’s establish a solid foundation by understanding what spheres and hemispheres actually are. A sphere is a perfectly round three-dimensional object where every point on its surface is equidistant from the center. Imagine a perfectly round ball, and you have a visual representation of a sphere. Now, imagine slicing this sphere precisely in half. The resulting half is called a hemisphere. It encompasses half of the sphere’s surface, with a flat circular base.
Formulas: The Key to Solving Surface Area Problems
The foundation of calculating surface area lies in understanding the applicable formulas. For a sphere, the magic formula is: Surface Area = 4πr², where ‘r’ represents the radius of the sphere. As for a hemisphere, the formula takes the form: Surface Area = 2πr² + πr². This formula incorporates the curved surface area (2πr²) and the area of the circular base (πr²). Keep these formulas handy as we move on to exploring the nuances of solving problems.
Working Through Worksheet Delights
Let’s bring our newfound knowledge to life with a practical example from a surface area worksheet. Imagine a question asks: “What is the surface area of a sphere with a radius of 5 centimeters?” Using the sphere’s surface area formula, we plug in the radius value: Surface Area = 4π(5 cm)² = 100π cm². Alternatively, if the question involves a hemisphere with a radius of 3 inches, we apply the hemisphere formula: Surface Area = 2π(3 inches)² + π(3 inches)² = 27π inches². Just like that, we’ve cracked the code!

Image: lessondbchoi123.z13.web.core.windows.net
Unveiling the Power of Practice
Like any skill, mastery of calculating surface area comes with practice. Worksheets provide a fantastic platform to hone these abilities. Each problem presents a unique scenario, allowing you to experiment with the formulas, solidify your understanding of the concepts, and build your confidence. Be it a sphere with a given diameter or a hemisphere with a specific radius, every problem you tackle strengthens your command over the topic.
Tips and Expert Advice for Surface Area Domination
Here’s a secret – conquering surface area worksheets isn’t just about memorizing formulas. It’s about strategic thinking and employing effective approaches to simplify the process. Firstly, ensure you have a clear understanding of the formulas for both spheres and hemispheres. Next, focus on breaking down complex problems into smaller, more manageable steps. If a question asks for the surface area of a hemisphere cut from a sphere of a certain radius, begin by picturing the scenario and then apply the appropriate formulas separately for the curved surface area and the circular base. Lastly, don’t hesitate to use visual aids such as diagrams or sketches to enhance your comprehension and make the calculations more intuitive.
Frequently Asked Questions
Q: What are the key differences between spheres and hemispheres?
A: A sphere is a fully round three-dimensional object with all points on its surface equidistant from the center. A hemisphere, on the other hand, is half of a sphere, with a flat circular base.
Q: How does understanding surface area relate to real-life situations?
A: Surface area calculations find practical applications in various fields. Architects use them to determine the amount of materials needed for building domes or spherical structures. Engineers rely on surface area calculations for optimizing the design of containers or storage tanks. And even in everyday life, knowing surface area helps us estimate how much paint is needed to cover a spherical object or how much fabric is required to make a spherical balloon.
Q: What are some common mistakes to avoid while solving surface area problems?
A: One common mistake is misinterpreting the formulas. Ensure you use the correct formula for spheres and hemispheres. Also, be careful with units of measurement – consistently stick to the same unit throughout the problem. Another tip is to avoid making careless errors during calculations, particularly when dealing with squaring or multiplying. Remember to review your answers meticulously.
Surface Area Sphere And Hemisphere Worksheet Answers
https://youtube.com/watch?v=5HTVDxrmQBc
The Concluding Note: Take Action!
As we wrap up our exploration of sphere and hemisphere surface area worksheets, remember that the key to mastering any skill lies in practice, and practice, and more practice! We’ve covered the formulas, tackled practical problems, and shed light on common pitfalls. Now, it’s your turn to take charge. Grab those worksheets, delve back into the formulas, and confidently solve those surface area challenges! And if you find yourself still pondering some of these exciting geometrical concepts, feel free to ask questions! We’re here to help you navigate the fascinating realm of spheres, hemispheres, and surface area calculations. The journey of understanding these concepts is both rewarding and fulfilling.
Are you interested in exploring deeper into the world of spheres, hemispheres and surface area problems? If so, let us know and we can delve into more complex calculations, real-life applications, and innovative problem-solving techniques!





